Error Correction Laboratory

Project Summary

Fault-tolerant quantum computers need to both protect quantum information from noise as well as allow computation on the protected information. For these computers to be scalable, quantum error correction (QEC) is essential. Broadly, the goals of QEC for quantum computing have two thrusts: good and efficient error correction, fault-tolerant logical operations. While codes have been constructed and studied with respect to these thrusts, a coherent theory of how the desired code structure for one thrust affects the other is lacking. Establishing this connection is both fundamentally important as well as practically relevant for developing scalable fault-tolerant quantum computers. In this project, we particularly study the promising class of codes called quantum low-density parity-check (QLDPC) codes and set out to understand the dynamics in its structure with respect to the two thrusts.

Project Outline

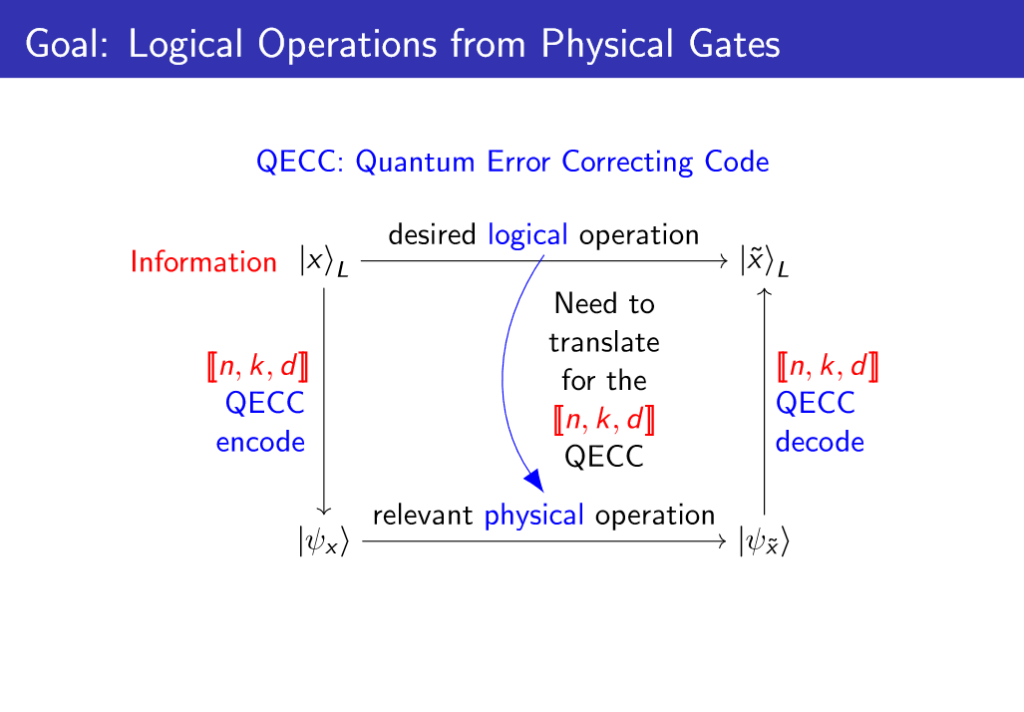
A quantum error correcting code (QECC) encodes few logical qubits of quantum information in many physical qubits developed in the lab. The larger mathematical space corresponding to these physical qubits contains redundancy on top of the encoded information, and this redundancy helps detect and correct errors on the data. While the information is encoded into these physical qubits, performing fault-tolerant computation on this information is tricky. We need to understand which quantum operations on the physical qubits induce which logical operations on the encoded (logical) qubits. Moreover, these quantum operations must be performed fault-tolerantly, i.e., an error in one part of the operation must not spread catastrophically to many physical qubits. These constraints impose structural conditions on the QECC, and they tend to be algebraic in nature.

On the other hand, when errors occur on the physical qubits in the lab, we need to make necessary measurements and fix these errors. Importantly, we must not allow the errors to accumulate and overwhelm the QECC. The need for performing effective detection and correction of errors imposes certain types of structural constraints on the code, e.g., the code should preferably have a representation as a sparse graph. However, imposing the sparse graph constraint might directly affect the feasibility of performing fault-tolerant logical operations. This dynamics between fault-tolerance and error-correction is only loosely understood in the literature. In this project, we focus on QLDPC codes, which have a sparse graph structure, and set out to understand how fault-tolerant logical operations can be performed on the code while retaining the ability to effectively correct errors. This pursuit leads to very interesting mathematical and computational problems that span algebra, finite geometry, graph theory, and algorithms.

Project References

N. Raveendran and B. Vasić, “Trapping sets of quantum LDPC codes,” Quantum 5, pp. 562, 2021

N. Raveendran and B. Vasić, “Trapping Set Analysis of Finite-Length Quantum LDPC Codes,” 2021 IEEE International Symposium on Information Theory (ISIT), 2021, pp. 1564-1569

J. Hu, Q. Liang and R. Calderbank, “Designing the quantum channels induced by diagonal gates,” arXiv preprint arXiv:2109.13481, 2021

N. Rengaswamy, R. Calderbank, M. Newman and H. D. Pfister, “Classical Coding Problem from Transversal T Gates,” 2020 IEEE International Symposium on Information Theory (ISIT), 2020, pp. 1891-1896

N. Rengaswamy, R. Calderbank, S. Kadhe and H. D. Pfister, “Logical Clifford Synthesis for Stabilizer Codes,” in IEEE Transactions on Quantum Engineering, vol. 1, pp. 1-17, 2020, Art no. 2501217

Grant: NSF Project 2106189